I would like your opinions on this youtube link.
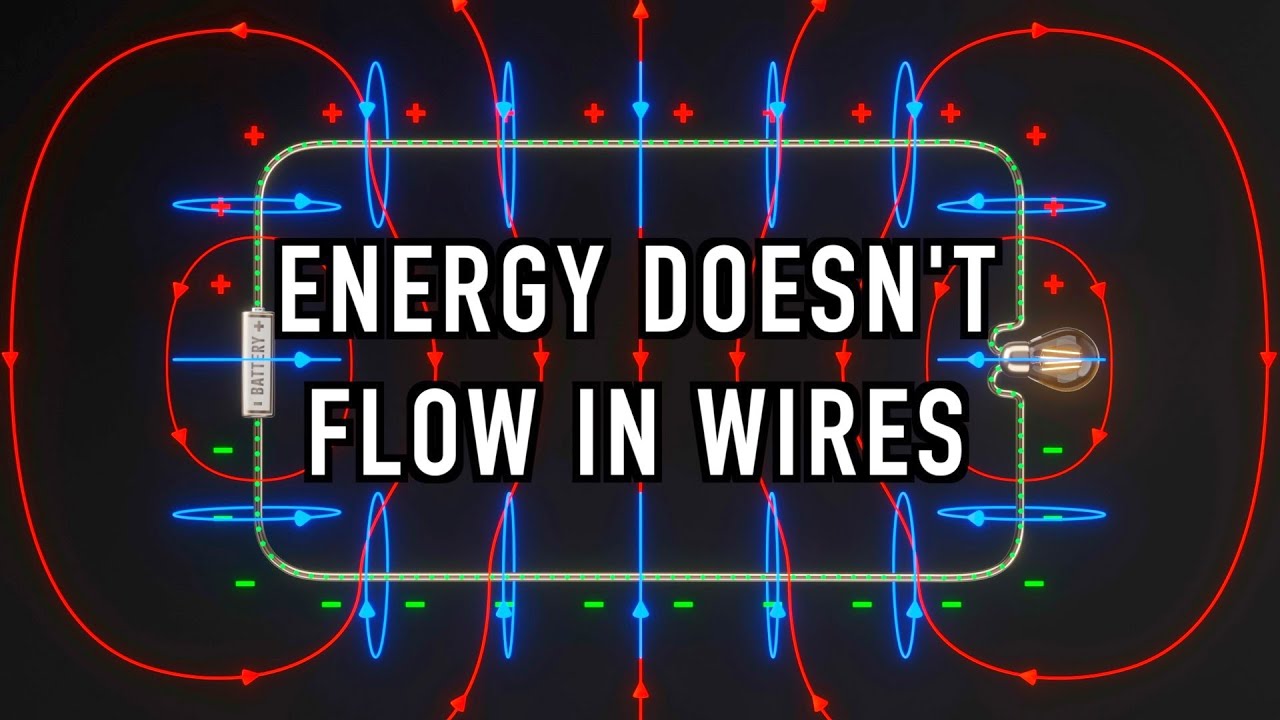
The author postulates that energy is transmitted not in the conductors, but in the field established around the conductors.
Thanks,
I didn’t watch the video, but electricity is a field composed of electric and magnetic energy, so that’s probably correct.
A useful model is Heaviside’s telegrapher’s equation; cf.
Note, you can’t simply eliminate the wire itself, unless you are building an antenna
Do shielded conductors have the field in place between the conductor and the shield?
I believe that is what one tries to achieve with coaxial cables.
Yeah. It’s been decades since I studied EM fields, but I seem to recall that the energy doesn’t flow in the interior of the wire. I’ll give the video a watch, though.
Indeed.
I observed the experiment in undergraduate school described in the video. Passing a signal through a long length of coax showed the signal to be traveling at something like .8C
That sounds like the statement that electric fields cannot extend into a “perfect” conductor, by Gauss’s law.
I have been stewing over this video for a while. It really annoyed me and is one of Derek’s poorer efforts. There have been quite a few follow-up videos from various quarters as well.
My view is that Derek has made a significant mess of this video. What he presents is both true and badly misleading. It took me a while to realise what he was really trying to say, and why. This alone underlines why the video is such an uncharacteristically poor one from him.
Very early on, Derek has his little demonstration with the chain in a tube as an analogue of electrical current, and then talks about power flow. He makes a critical observation that if you miss (and I did at first) makes the rest of the video puzzling and maddening. He claims that people are taught that electrons have electrical potential. He then goes on to say that people are taught (and moreover, when he taught electrical theory that he taught) that flow of this electrical potential in wires is how energy is transmitted. This idea is the lie he is trying to address. Which is reasonable, it is terrible, and just plain flatly wrong. Electrons don’t have electrical potential. They have charge. (I had never come across the idea that anyone would have taught electric flow the way Derek says he taught people. I sure as heck, even at high school, was never so taught.)
Where it all goes south is when he tries to show us that power is transferred via the electrostatic and magnetic fields. Here he proposes a maddeningly stupid and misleading experiment.
The answer is that a small amount of power will indeed be transmitted across the gap in about 3ns. However we can calculate how much power is transmitted in this manner, and it isn’t much. What Derek implies is that this is the mechanism by which the full power of the source is available to the lamp. Which it is not. That power will traverse the wires and be available once the full length of the wires are traversed.
Some quick thought experiments over and above his basic one.
- Q. If instead of two legs in opposite directions, the two legs overlaid one another on one side, would the lamp light?
- A. No.
This alone requires pause for thought. Derek goes to huge lengths to convince the viewer that electrical power doesn’t go down the wires, but simple rearrangement of the wires breaks his demonstration.
- Q. If the legs had a break in their far ends, would the lamp light?
- A. Yes.
For the purposes of Derek’s question, the lamp would light after 3ns. It would also go out again. So again, it is clear that something is amiss.
Derek proposes legs with wires 1 metre apart. If we assume a reasonable size for the wires, say 1mm radius, we can calculate the characteristic impedance of what is a transmission line. It is about 800Ω. Since there are two legs, we have about 1600Ω impedance between the battery and the lamp. That tells us the maximum power a 12 volt battery, such as Derek shows us in his experiment, can deliver to the lamp.
About 0.1 Watts. This will not light an ordinary light bulb. It will light a tiny LED. Derek posits an ideal light bulb that lights as soon as energy is available, but he does not demand a light bulb that breaks conservation of energy. There his answer is misleading. In particular, the lamp he uses in his demonstration would not light.
IMHO this is why his presentation is so misleading. He goes on about the Poynting vector, and power delivery, and talks about how power distribution networks operate, and how power does not flow in wires. The casual viewer is left believing that Derek has told them that electron flow in wires has nothing to do with energy flow in wires, and that the actual flow of energy occurs in the fields around the wires, and is able to jump across the wires, taking the shortest path.
You’re probably right
I am skeptical of this video. Maybe it was just a poor explanation of a correct concept. But it seemed to lean in very hard on what appears mostly incorrect.
One thing about all the energy being in the fields. Then why does the wire have to be thicker for more energy at a particular voltage? Too small a wire will actually melt if too much current is drawn through it.
As frequency increases, skin effect increases. Resistance increases. But why would that be an issue if all the energy was in the fields?
I do agree that there are fields around a conductor. Basic electric fact. But not getting his explanation.
My first thought was that a conductor becomes warm. There is power created with current flowing through the resistance of the conductor.
But it’s not necessary for a conductor to become warm in order to “carry” electrical power. Wires made of a superconductor would carry power very nicely, and the wires would not get warm.
Surely.
I haven’t seen the video yet but wanted to point out that as frequency increases more and more of the current flows on the surface of the wire, not the interior.
When the Space Station was proposing to use 20,000Hz power all the wires we used were wide flat ribbons of foil, basically. No more round wires. It was proposed that this was safer as current would flow on the outside of your body instead of through the heart, etc. My buddy Dan demonstrated this when he backed into an exposed terminal and lit himself up.
It is true that the Poynting vector (which is what describes the flow of energy) mostly exists outside of the conductor itself (aside from some small amount, in a non-superconductor, representing the flow into the wire itself which heats it up).
I’m not interested in watching a 15-minute video to see if he explains this well, though.
I was looking forward to your discussion.
Huzzah for my thirty year old memories
Clarifying what I wrote above.
The “No” applies to the idea that the lamp will light after 3ns. It won’t. It will however light up after the power comes down the wires.
There are a lot of interesting traps in the experimental setup Derek proposes. I don’t think he intended a lot of them. It underlines the dangers of ideal elements in an experiment that is attempting to describe the real world. He allows for wires with zero resistance. Of course this is intended to avoid worrying about losses in a one light second length of wire. But it has the side effect of excluding the fields from the interior of the wire. I don’t think Derek intended a “Ha ha, got ya” answer because of this, but it complicates the answer and the discussion.
The setup acts as a transmission line, and Derek is talking about immediate power delivery. Yet he glosses over transient effects and transmission line activity, whilst the real answer is going to be a quite complicated mess as the line reflects energy and rings and radiates power into space. His only question is “when does the lamp light”, with no discussion about what follows. The viewer is left with the impression that a steady state is achieved as soon as the lamp first lights, and this steady state is what describes steady state power delivery. This is especially bad as the very limited power delivery available in the first moment is most definitely not the steady state. To understand how the system would act in reality requires a lot more information about the components. And this means stepping back from simplified ideal components.
The hydraulic analogy isn’t perfect but this is one thing that it gets exactly right. The electrons don’t carry energy any more than oil carries energy in a hydraulic loop. The fact that the oil circulates back to the pump doesn’t cause any problems with energy flow, because it’s the pressure that transmits energy, and a pressure drop is how a device takes energy from a circuit.
Likewise, electrical circuits have electrical potential, which corresponds to pressure. Devices consume energy when there is a drop in potential in the same direction as the current.
The electromagnetic fields can’t be ignored of course, but they’re mostly only relevant outside the steady-state case.