I have a basic understanding of fusion, basically a few atoms of Hydrogen (for example) fuse together to create Helium.
Sounds painless enough, but why does the act of fusing atoms create such enormous amounts of energy?
I have a basic understanding of fusion, basically a few atoms of Hydrogen (for example) fuse together to create Helium.
Sounds painless enough, but why does the act of fusing atoms create such enormous amounts of energy?
It doesn’t create the energy. The energy was there all along. In principle, it’s no different than coal releasing energy when you burn it.
Except, of course, that fusion releases a lot more energy than coal. That’s basically because the energy release in burning coal is associated with the electromagnetic force that holds molecules together, but the energy in fusion is associated with the strong nuclear force, which holds the nuclei of atoms together. And the strong nuclear force is, as its name suggests, much stronger than electromagnetism.
E=MC Squared. (I don’t know how to do a superscript here, or even if you can.) Energy released in a fusion reaction equals the mass of the hydrogen atoms (minus the mass of the created helium atoms, in this case) times the speed of light squared. Really small number times really, really big number=big number.
Like this: E=MC[sup]2[/sup] (for subscript, replace the sup with sub)
In nuclear fusion, nuclei are forced together under very high pressure and temperature such that the nuclei combine. This results in excess ‘binding energy’, analogous to the energy in electrochemical bonds but many orders of magnitude stronger, which is released in the form of kinetic energy of the resulting products, energetic photons (X-ray or gamma ray radiation), and depending on the reaction, one or more neutrons or protons. The most viable forms of fusion for terrestrial power production or weapons involves isotopes of hydrogen (deuterium and tritium in D-D and D-T reactions), which fuse to tritium or helium, but higher atomic number fusion does occur in stars (“helium burning” and the CNO cycle. (Thermonuclear weapons actually use neutron production from a fission Primary to “breed” tritium from lithium by absorption and decay because of the difficulty of storing and replacing large amounts of unstable tritium, which has a half-life of 12.3 years.)
Far from being ‘painless’, creating the conditions in which fusion reactions will occur in quantity requires an environment not naturally seen on Earth, and in fact for the ‘prompt’ fusion seen in thermonuclear weapons the temperatures exceed that in the core of our Sun, or any star short of one becoming a nova. We have yet to create such conditions in a sustained fashion such that the energy yield exceeds the energy required to maintain confinement of the plasma in a steady-state condition. What seemed like an energy production technology just around the corner circa 1950 has eluded scientists and engineers for seven decades because of the complex interactions in energetic plasmas.
Although fusion power production is often described as ‘clean’ (not producing pollutants like petrocarbons or coal, or radioactive waste like fission) the most viable fusion reactions product neutron radiation, whch can embrittle or activate (make radioactive) many common materials, so even if a suitable method could be developed for steady-state confinement there are still major technical challenges, notwithstanding figuring how how to convert he energy in high temperature plasma to something usable without disrupting the plasma. There are several interconnected programs working on various aspects of fusion power technology, but it is quite challenging for the state of the art in materials and simulation.
Stranger
The Binding energy for elements up to Iron progressively have a lower potential energy than its constituent parts.
In effect when you fuse these elements it is like a ball rolling down hill until you hit Iron.
Here is a chart that will show this better than a wall of text.
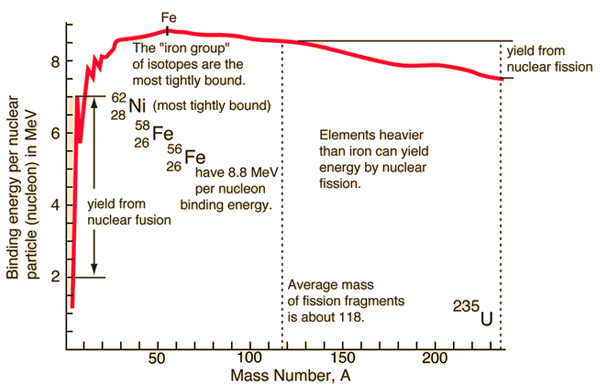
Note this is a simplification, the total Energy and Rest mass are dependent not only of the parts that they are made of but how they are arranged.
As an Example, a molecule of water has less mass than two free hydrogen atoms and an oxygen atom. This is observable when you burn a log or drive your car, the exothermic energy is released as the parts assemble themselves into a lower energy state.
Note this is not universal, as you can see in the chart that Fusion above Iron requires more energy than it releases. This is also observable greater difference in the Proton which has a mass ~100 times greater than it’s valance quarks would have on their own.
It may be helpful to note that E = MC^2 is simplified, and that we should consider the more complete formula e2=(mc2)2+ (pc)2 Where ‘p’ is the potential energy.
In the case of a Proton it has a massive amount of stored(potential) energy, but a Helium atom has less stored energy than a Hydrogen atom.
No, the p in that formula is the momentum. Since all particles in the reactions we’re talking about start and end at fairly low velocities, the momentum is not particularly significant. The potential energy is the mass.
As the strait dope lacks the mathtex support I will say i was simplifying.
Correct me if wrong but in high-energy physics, the electronvolt is commonly used as a unit of momentum.
Sure in the quantum world, momentum p is replaced by the momentum operator, and those values are far from trivial, thus the huge difference between the much smaller mass of the valance quarks and the much more massive baryon.
This is a simplification but the total energy of that system is comprised of.
In the case of the proton the rest masses of the constituent parts is lower than the composite system, and the kinetic energy is a significant portion of the rest mass of that total system.
While crossing domains and touching several areas of unsettled science this is also compatible with my analogy, even if not the best way to describe it.
Consider water having less mass than the constituent parts, the water particle has a tendency to remain localised in one or more regions of space, and as a momentum is a vector it can be negative, which is the typical way I have seen it described.
While still a simplification, the heat produced when a water molecule is produced can be thought of as kinetic energy or momentum too. Sure it is a spherical cow, but what isn’t in physics.
Anyway, as far as the “release” of energy from fission, Kinetic energy’s scalar and momentum vector being and/if/when they are reduced to a common term etc… is a distraction.
The point is that E=MC^2 confuses lots of people who don’t have a very firm grasp on the Mass-Energy equivalence, because it abstracts the idea that it is not just what parts make up a collection but also how they are arranged that influences the big M above. And to be clear I used the term potential because it is the most common term used when teaching about the ‘mass defect’.
I do want to point out that 99% of the mass of a proton is in that bound energy, and not in the quarks’ rest mass, that is absolutely significant.
I just Googled to be sure; it is a measure of energy.
But to be fair, people drop the /c for both momentum and mass
Energy = (mass)(length)^2/(time)^2
Divided by (length)/(time) =
(mass)(length)/(time) or Momentum
Divided by (length)/(time) =
(mass) or Mass
Note how the above (ignoring momentum) can be written as m = E/c2, which is E=mc^2?
Chemical bonding is arranging elements a lower state release energy, which can be viewed as the momentum portion.
Fusion, via the strong force (below Iron) is arranging elements a lower state release energy, which can be viewed as the momentum portion.
It is the momentum portion of the above break down that is often released as heat, photons etc…
While part of the confusion is caused by the convention of dropping the ‘c’ aspect in what would be properly called Energy(MeV), Momentum(MeV/c), and mass(MeV/c2) it is assume that E=mc^2 is understood so they all tend to just use MeV.
That is exactly the misunderstanding I was trying to address.
A simple balancing of units tell you that (energy squared) cannot equal (energy squared)x(velocity squared).
I am confused about what point you are making here, as I didn’t make that claim.
let me provide a cite for the page I cargo culted the ASCII formulas from. But I do wish this site had mathtex.
Each component of that equation has the units of (energy squared). If “p” is energy, the units don’t balance. Your link has nothing to do with the equation we are discussing in which “p” is momentum. Physicists routinely use “p” to mean momentum, and if they meant “potential energy”, they’d use E[sub]P[/sub]. But the “p” in the equation is momentum no matter what symbols are used. Saying that it’s “potential energy” is like saying “m” must be “momentum” because momentum begins with m.
Yes, you can measure energy, momentum, and mass all in the same units if you’re implicitly defining c as 1. What does that have to do with the incorrect fact you posted?
It’s common in physics to set ħ, c = 1. Potential energy and momentum, on the other hand, are very different things, and the standard equation E[SUP]2[/SUP] = m[SUP]2[/SUP] + p[SUP]2[/SUP] unambiguously involves the latter. (It’s also common to use p to denote the 4-momentum (E, p[SUB]1[/SUB], p[SUB]2[/SUB], p[SUB]3[/SUB]), which conveniently makes the equation above p[SUP]2[/SUP] = m[SUP]2[/SUP].)
Momentum is not energy. (Classical 3-) momentum is a vector; energy is a scalar. Photons carry momentum (they have to, since they’re massless); heat is a scalar.
I don’t know what you’re trying to say here.
Winding back, for the OP. I think for anyone coming to fusion with a very basic understanding, Chronos made the most important point very early on.
This is really key, and not much appreciated. People have the habit of talking about a mass imbalance and then invoking E= mc[sup]2[/sup] to explain that this mass is now that much energy. But in a fundamental way, that is backwards. And in a surprising way.
We all learn that the nucleus is make up of proton and neutrons, and the number of protons defines what sort of element we have. The first puzzle that scientists had was to explain why all the positively charged protons didn’t just repel one another, and nuclei didn’t just fly apart. And the answer was the presence of neutrons, and what was at first a puzzling way. You need neutrons to bind with the protons, and they will form a stable atomic nucleus, As a rough approximation, about the same number of neutrons and protons. (Slight variations in the number of neutrons giving us that different isotopes of an element.) Just how protons and neutrons held together was the next puzzle. That was sort of solved when additional particles were discovered (mesons) that included particles that the protons and neutrons would exchange in a little dance that kept them close to one another. So they had a “strong” nuclear force medicated by these pions (one of the sorts of mesons discovered). Then along cam Gell-Mann and co, and they described how protons, neutrons, mesons, and lot of other stuff, was made of quarks. So now we didn’t have a strong force anymore, but rather the special force that quarks are bound by - the colour force. Mostly the colour force is holding the quarks together inside protons, neutrons etc, but a little of the force is seen outside these particles, and that gets called the residual atomic force - and is what we used to call the strong force. But back to the insides. Something quite astounding was discovered. The energy binding quarks together is colossal. Staggering. It turns out the the quarks we worry about here are actually reasonably light particles as they go, and large fraction of the mass of a proton or neutron is not the masses of the quarks, but the energy that is binding those quarks together. E = mc[sup]2[/sup] isn’t just a formula for converting energy to mass and vice versa, it tells us how much mass an existing collection of energy has. This huge binding energy inside protons and neutrons gives these particles most of their mass. Which means it give us most of our mass.
Now when we rearrange a few atoms (and maybe some spare neutrons) we find that the energy needed to bind all the resulting neutrons and protons together in the new atom, will usually not be the same as was needed to bind together the original atoms. As described earlier, light and heavy atoms need more energy to hold themselves together than mid-range atoms, with iron being the element right n the middle that needs the least binding energy to hold its bits together. This energy binding the protons and neutrons together, is as we saw, just the colour force, but in the form as it is seem outside the individual protons and neutrons as the residual atomic force. And again, this binding energy has mass, via E = mc[sup]2[/sup] So, any way we can rearrange atomic nuclei that results in a new stable configuration (ie a balance of protons and neutrons) is going to change the amount binding energy present. And if you do so in such a way as form wherever you start your end product is closer to iron than when you started, you will have some binding energy left over. This also tells you that you final produce will be lighter by the amount of energy you had left over.
It turns out that the single biggest jump in binding energy of from a hydrogen atom to make helium. So there is a potential win here. But as described above, making it happen is really hard. Overcoming the repulsive forces between those various positively charged nuclei is not trivial, and needs a lot of energy in the first place. You need to have the atoms moving really fast (ie very hot) and under a lot of pressure. Either that, or you need to be in no hurry. Worse, you need you end product (helium) to have a stable configuration - whihc means you need a couple of neutrons to add to your protons. On Earth we tend to start with hydrogen that already has a neutron, so our reaction balances. This means we need to use Deuterium (aka heavy hydrogen). Otherwise we would be waiting even longer for a random passing neutron to come past at the exact right moment. Which is fine if you are in the middle of a star, and have billions of years to waste, but not so good if you are trying to make electricity or wipe out a nearby nation.
But under it all. Both fusion and fission are about working out how to convince various elements to re-arrange their constituent protons and neutrons in such a way that a tiny smidgen of the internal binding energy is left over. The amount of energy that is actually there is enormous. So much so it is responsible for the majority of our mass. We are just looking for the crumbs off the table.
Beautiful.
Looking at the link in rat avatar’s post #6 we see the left half of that diagram is way, way steeper than the right half. Simplifying a bunch, that’s the difference between the energy available from a fission reactor or bomb versus that available from a fusion reactor or bomb. Hence the interest in developing ways to harness the left half of the diagram.
Thanks. It really needs some serious proof reading and spell checking. I wrote it in too much of a hurry, and now I see some terrible typos.