Naah, it has nothing to do with ED drugs. The joke is simply the fact that [uncompressed] helium is lighter than air.
The helium is compressed, so divers don’t float away, but do you know what other property of helium affects divers?
It gives them a high pitched voice. To the extent that surface crew sometimes uses pitch correctors so they can understand the diver’s speech (in cases where they’re using a full face mask and can speak at all).
And of course it fits in with the cartoon trope of helium not merely being buoyant in air but some sort of magically antigravity substance.
Compressed helium should float even harder, right?
Screw the vacuum zeppelins; we should be building compressed helium zeppelins. Heck, go all the way and use liquified hydrogen.
It’s too cold to burn. So much safer than the gaseous kind. No more Hindenburgs for us.
Stands to reason!
Better yet: liquified hot air!
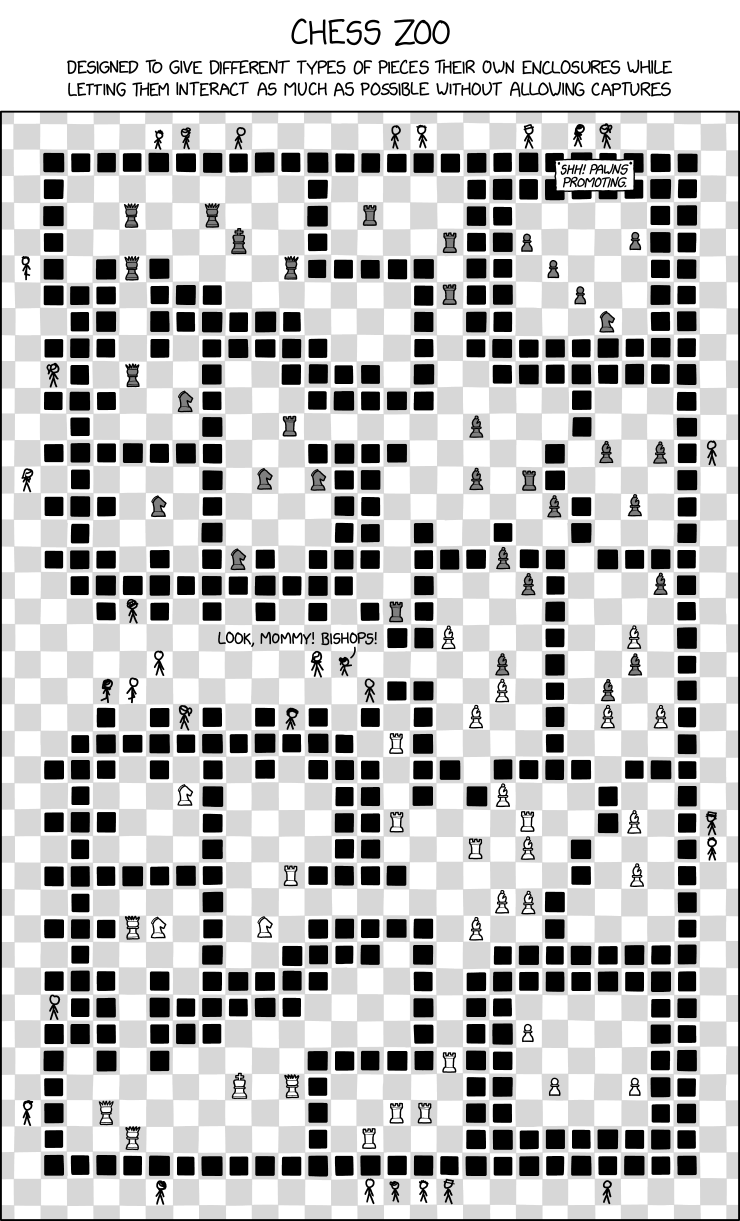
“The zoo takes special care to keep kings separated from opposite-color pieces as part of their conservation program to prevent mating in captivity.”
That’s an interesting bit of imagination. Some of his bursts of inspiration come from gosh-knows-where. But I’d like to visit.
With the exception of the section at 3-o’clock with both colors of bishops in the same enclosure, but each trapped on their own color squares, there’s not much opportunity for interaction between the black and white pieces.
Which led me to wonder about whether fratricide is allowed, expected, or prevented by the peice’s natures. Kinda like at a real zoo you might have 2 kinds of antelope living in the same enclosure, but not also with wolves.
At upper right where it says “Pawns promoting” I noticed that, as best my (very) limited chess skills can prove, the knight could wander forever in that space and never capture any of the pawns. Until they move. Which suggests that if they are to promote successfully, the knight had better not be interested in eating them.
I also liked the rook-and-bishop proof barrier at the lower right of the people’s central viewing area. Good visibility into the enclosure but the peices can’t escape.
I did not find a black king. Although each color has several queens. I predict palace intrigue.
Top left enclosure
I didn’t see a means of letting (same-color) bishops and knights interact. It could be done with a 4-wide wall with a diagonal slice through it. Maybe there’s a more efficient way.
No way to do it with a 3-wide wall. No matter what the configuration, if the centerline of the wall contains a gap, knights can get through. But bishops require a gap to get through.
But a 4-wide wall in a checkerboard configuration will work. Knights can jump to the first or second row but no further. Bishops (of one color) can obviously pass through easily. Not sure yet if there’s anything lower density than 50%, but I suspect not.
I was looking at the area just south of the people entrance. There’s about a 4x4 area now containing a single white knight. The ++++ nature of the two exterior walls prevent the knight from getting out in public while still providing the people a decent look through. But …
That same enclosure is walled off from the enclosures to its south and east by just straight single-layer walls. Which knights can vault easily. Those other two enclosures have a queen, a rook, and 2 other knights. Which means the knights have a little sanctuary from the other pieces. OTOH, the queen and rooks can slip through the 1x4 passageways to the next enclosures to south and east, while the knights cannot. So their sanctuaries are larger.
There is a lot of depth to this zoo’s construction.
Quoting myself for context:
Turns out that’s wrong. There is a “knight’s tour” of a 5x5 board: Knights-Tour-Animation - Knight’s tour - Wikipedia from
Of course being able to visit every square just once is overkill compared to the question of whether every square can be visited allowing repetition.
Yeah, you have to have a pretty small enclosure (or a pretty small nook off of a larger enclosure) for knights not to be able to visit every space. From any square in a rectangle at least 3x4, a knight can reach any other square in that rectangle.
If I read correctly, not possible on a 4 X4 board.
Or are you saying a non-square board of at least 3x4? I believe that is correct.
Brian
“all squares are rectangles, but not all rectangles are square”
I don’t know if a tour (visiting each square exactly once and then returning to the start) is possible on a 4x4 board, but a knight can certainly reach any of the spots from any other. On a board labeled A-D and 1-4, which two spots do you think are cut off from each other?
All I know is in this section (Number of Tours) of the Wikipedia it has 0 for a 4x4 board:
Also, above that there is this:
Cull et al. and Conrad et al. proved that on any rectangular board whose smaller dimension is at least 5, there is a (possibly open) knight’s tour.[4][11] For any m × n board with m ≤ n, a knight’s tour is always possible unless one or more of these three conditions are met:
see also
https://www.mayhematics.com/t/oc.htm
4×4 board
No complete 4×4 tour is possible. There are four half-tours, but no two can be combined to give a full tour since their inner ends are on the 1st and 4th files which have no connecting move. Each is composed of one ‘square’ and one ‘diamond’.
Brian
But knight’s tours have a restriction (no square can be repeated) that can be ignored if we’re just trying to see if you can get from any square to any other.
Ah, my mistake — as far as I can tell you can get from any square to any other in a 4x4 grid . Proof is left as an exercise for the reader ![]()
Brian
Pretty easy to see that a knight’s tour on a 4x4 is impossible. Consider opposite corner squares. They only have two exit routes each, and in a knight’s tour every square must have exactly one entrance route and one exit. So the routes from the corners are totally determined, and from opposite corners they connect in the middle (making a narrow diamond). Since those middle squares now also have two determined routes, there’s no way to enter or exit that loop and get to the rest of the board.
